Mental Arithmetic – Square roots
Score
New Quiz
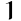
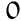
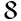

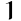
Players
The square root is the fundamental arithmetic operation of finding a number that, multiplied by itself, yields a specific quantity. It is represented by the symbol “√” and, when applied, indicates the number which, squared, produces the initial number.
For example, in the operation √16 = 4, 4 is the number which, multiplied by itself (4 x 4), gives 16.
The importance of the square root in mental arithmetic should not be underestimated. It is used on a daily basis in many situations, such as solving problems in geometry, managing personal finances or analyzing statistical data. Knowing how to calculate square roots quickly and accurately is therefore a valuable skill.
In physics, for example, the square root is essential for determining values such as mean velocity, kinetic energy and other derived measurements. When analyzing data, it is often necessary to extract square roots to normalize results or perform statistical calculations. Similarly, the square root plays a key role in understanding measurement scales and proportions.
More than a simple mathematical operation, the square root is an essential tool for navigating many practical aspects of everyday life. Its role in mental arithmetic is crucial, enabling us to make informed decisions, solve everyday problems and manage resources efficiently.
In mathematics, and more specifically in the field of square roots, the term “root” is of particular importance. It refers to the number obtained after extracting the square root of another number.
To illustrate this concept, let’s take a simple example: if you have the number 25, the square root of 25 is 5, because 5 multiplied by itself gives 25. In other words, the “root” represents the number which, multiplied by itself, gives the initial number.
The “root” applies not only in simple scenarios, but also in more complex contexts. For example, in the case of the area of a square, if the area is 36 square units, the length of each side of the square is the square root of 36, i.e. 6 units.
This concept is essential because it provides a quantitative measure that can be used in many everyday and professional situations, whether calculating dimensions, analyzing data or solving equations. The “root” is therefore an essential mathematical tool, offering a clear and quantifiable perspective on the results derived from extracting square roots.
The square root, an essential mathematical operation, is omnipresent in many everyday and professional activities. The efficiency of this task can often require faster execution, especially with complex numbers. Fortunately, there are techniques to speed up the procedure while preserving accuracy.
The estimation method is an effective approach that involves finding two numbers between which the square root lies, then refining the estimate. For example, to find the square root of 50, you know that it lies between 7 (7×7=49) and 8 (8×8=64). By refining, you can estimate that the square root of 50 is around 7.1.
Another strategy is to use square root tables or specialized calculators to quickly and accurately find the square roots of complex numbers. Although this method is more technical, it is effective for simplifying calculations involving large numbers. For example, to find the square root of 1681, you can use a calculator to quickly determine that it equals 41.
Regular practice of these techniques is essential to their mastery. Repetition makes these methods intuitive, which facilitates the rapid calculation of square roots. It’s important to note that increasing speed is the result of continuous practice. Accuracy, however, must not be compromised. It is advisable to check results regularly, especially during the learning phase.